The Ultimate Guide To Understanding Seven Sided Regular Polygon: Geometry Simplified
A seven-sided regular polygon is defined by its seven equal sides and angles, creating a perfect balance that is both visually appealing and mathematically significant. Whether you’re a student learning geometry or simply curious about shapes, understanding this polygon can open doors to deeper insights into the world of mathematics. Diving deeper into its characteristics, the seven-sided regular polygon is more than just a geometric figure. It is a symbol of precision and balance, often used in architecture, art, and design to create visually striking patterns. Its internal angles, side lengths, and symmetry are governed by mathematical principles that have fascinated scholars for centuries. Beyond its theoretical importance, the heptagon also finds practical applications in fields such as engineering and computer graphics, making it a versatile and essential shape to study. In this article, we will explore the seven-sided regular polygon in detail, covering its properties, construction methods, real-world applications, and much more. By the end of this guide, you will have a comprehensive understanding of this fascinating shape and its significance in both theoretical and practical contexts. Whether you’re here to satisfy your curiosity or enhance your knowledge, this article promises to deliver valuable insights into the world of heptagons.
Table of Contents
- What is a Seven Sided Regular Polygon?
- How to Calculate the Angles of a Heptagon?
- Why is the Heptagon So Rare in Nature?
- Construction of a Seven Sided Regular Polygon
- Applications of Heptagons in Real Life
- Seven Sided Regular Polygon in Art and Design
- How to Draw a Perfect Heptagon?
- Frequently Asked Questions About Heptagons
What is a Seven Sided Regular Polygon?
A seven-sided regular polygon, commonly referred to as a heptagon, is a two-dimensional geometric figure with seven equal sides and seven equal angles. This polygon is classified as "regular" because all its sides and angles are congruent, creating a perfectly symmetrical shape. The term "heptagon" is derived from the Greek words "hepta," meaning seven, and "gonia," meaning angle, which aptly describes its defining characteristics.
To better understand the heptagon, let’s break down its properties. A regular heptagon has internal angles that each measure approximately 128.57 degrees, adding up to a total of 900 degrees for all seven angles combined. The formula for calculating the sum of internal angles in any polygon is (n-2) × 180, where "n" represents the number of sides. For a heptagon, substituting n=7 gives us (7-2) × 180 = 900 degrees. This mathematical precision makes the heptagon a favorite among mathematicians and geometry enthusiasts.
Read also:Scarlett Johansson Height And Weight A Comprehensive Guide To Her Life And Career
What sets the seven-sided regular polygon apart from other polygons is its unique symmetry. Unlike simpler shapes like triangles or squares, the heptagon’s seven sides create a more complex and visually appealing structure. This complexity also makes it a challenging shape to construct using traditional tools like a compass and straightedge, which adds to its allure. Whether you’re studying geometry or exploring its applications, the heptagon offers a rich field of study and discovery.
Key Features of a Heptagon
- Equal Sides: All seven sides are of the same length.
- Equal Angles: Each internal angle measures approximately 128.57 degrees.
- Symmetry: The heptagon has rotational and reflective symmetry.
- Diagonals: A heptagon has 14 diagonals connecting its vertices.
Why Study Heptagons?
Understanding the seven-sided regular polygon is not just an academic exercise; it has practical implications. Architects and designers often use heptagons to create aesthetically pleasing patterns and structures. Additionally, its mathematical properties are studied in fields like computer graphics and engineering, where precision is paramount. By mastering the heptagon, you gain insights into both theoretical and applied geometry.
How to Calculate the Angles of a Heptagon?
Calculating the angles of a seven-sided regular polygon is a fundamental skill in geometry. The process involves using mathematical formulas to determine both the internal and external angles of the heptagon. Let’s explore how this is done step by step.
The formula for finding the sum of internal angles in any polygon is (n-2) × 180, where "n" is the number of sides. For a heptagon, substituting n=7 gives us (7-2) × 180 = 900 degrees. To find the measure of each internal angle, divide the total sum by the number of angles, which is also 7. Therefore, each internal angle of a regular heptagon measures 900 ÷ 7 ≈ 128.57 degrees.
What about the external angles? The external angle of a polygon is the supplementary angle to its internal angle, meaning it adds up to 180 degrees. For a heptagon, each external angle measures 180 - 128.57 ≈ 51.43 degrees. These calculations are essential for understanding the geometric properties of the heptagon and its role in various applications.
Step-by-Step Guide to Angle Calculation
- Determine the number of sides (n) in the polygon. For a heptagon, n=7.
- Use the formula (n-2) × 180 to find the sum of internal angles.
- Divide the total sum by the number of angles to find the measure of each internal angle.
- Calculate the external angle by subtracting the internal angle from 180 degrees.
Practical Applications of Angle Calculations
Knowing how to calculate the angles of a heptagon is not just theoretical; it has practical uses. For instance, architects use these calculations to design buildings with heptagonal floor plans, ensuring structural stability and aesthetic appeal. Similarly, engineers rely on these principles when creating mechanical components or designing algorithms for computer graphics. Understanding these calculations empowers you to apply geometric principles in real-world scenarios.
Read also:Mastering Remote Iot Vpc Ssh A Comprehensive Guide To Secure Connectivity
Why is the Heptagon So Rare in Nature?
Have you ever wondered why the seven-sided regular polygon is so rarely found in the natural world? Unlike hexagons, which are abundant in honeycombs and snowflakes, or pentagons, which appear in starfish and flowers, the heptagon seems to be conspicuously absent in nature. This rarity can be attributed to several factors, including the laws of physics, evolutionary biology, and structural efficiency.
One reason for the heptagon’s scarcity is its geometric inefficiency. Shapes like hexagons and triangles are more efficient at tiling a plane without leaving gaps, which is why they are commonly found in natural structures. For example, bees use hexagonal cells in honeycombs because this shape maximizes space and minimizes material usage. In contrast, a heptagon cannot tile a plane without creating gaps or overlaps, making it less practical for natural formations.
Another factor is the complexity of the heptagon’s angles. The internal angles of a heptagon (approximately 128.57 degrees) are not conducive to forming stable structures in nature. Most natural shapes evolve to optimize strength and stability, and simpler shapes like triangles or hexagons are better suited for this purpose. This doesn’t mean that heptagons are entirely absent, but their occurrence is rare and often coincidental rather than intentional.
Examples of Heptagons in Nature
- Giant’s Causeway: This natural rock formation in Northern Ireland features some heptagonal columns, though they are not perfectly regular.
- Crystal Structures: Certain minerals may exhibit heptagonal patterns due to their molecular arrangements.
Why Study Rare Shapes Like the Heptagon?
Even though the seven-sided regular polygon is rare in nature, studying it provides valuable insights into geometry and symmetry. By understanding why certain shapes are more prevalent than others, we can appreciate the underlying principles that govern natural and man-made structures. This knowledge can inspire innovations in fields like architecture, biology, and materials science.
Construction of a Seven Sided Regular Polygon
Constructing a seven-sided regular polygon, or heptagon, is a fascinating challenge that requires precision and understanding of geometric principles. Unlike simpler shapes like squares or triangles, the heptagon cannot be constructed perfectly using only a compass and straightedge due to its irrational angles. However, there are methods to approximate its construction with remarkable accuracy.
To begin, draw a circle using a compass. This circle will serve as the circumcircle of the heptagon, meaning all its vertices will lie on the circle’s circumference. Next, divide the circle into seven equal arcs. Since 360 degrees divided by 7 is approximately 51.43 degrees, you can use a protractor to mark these angles around the circle. Connecting these points with straight lines will give you an approximate heptagon.
For a more precise construction, you can use trigonometric calculations or software tools like CAD programs. These methods allow you to achieve a high level of accuracy, which is essential for applications in engineering and design. While the traditional compass-and-straightedge method has its limitations, modern techniques make it easier than ever to construct a near-perfect heptagon.
Tools for Constructing a Heptagon
- Compass: Used to draw the circumcircle.
- Straightedge: Helps in drawing straight lines between points.
- Protractor: Useful for measuring and marking angles.
- Trigonometric Calculations: For precise measurements and constructions.
Why is Heptagon Construction Challenging?
The challenge in constructing a heptagon lies in its irrational angles, which cannot be expressed as simple fractions of a circle. This makes it impossible to divide the circle into seven equal parts using only a compass and straightedge. However, with modern tools and techniques, this challenge can be overcome, allowing us to create accurate representations of this intriguing shape.
Applications of Heptagons in Real Life
While the seven-sided regular polygon may seem like a purely theoretical concept, it has numerous practical applications in various fields. From architecture to technology, the heptagon’s unique properties make it a valuable shape for solving real-world problems. Let’s explore some of the most notable applications of this fascinating polygon.
In architecture, the heptagon is often used to design visually striking buildings and structures. Its symmetry and balance make it an excellent choice for creating aesthetically pleasing floor plans and facades. For example, the design of certain modern stadiums incorporates heptagonal shapes to optimize seating arrangements and ensure unobstructed views for spectators. Similarly, heptagonal patterns are used in tiling and decorative elements to add a touch of elegance and sophistication.
In the field of engineering, the heptagon plays a role in designing mechanical components and systems. Its geometric properties are studied to create efficient algorithms for computer graphics and simulations. For instance, game developers use heptagonal shapes to model objects and environments, ensuring realistic and immersive experiences. Additionally, the heptagon’s symmetry is leveraged in robotics to design balanced and stable structures.
Heptagons in Technology
- Computer Graphics: Used in modeling and rendering 3D objects.
- Robotics: Helps in designing balanced and stable robotic structures.
- Game Development: Enhances realism in virtual environments.
Why Are Heptagons Valuable in Practical Applications?
The seven-sided regular polygon’s unique
How Much Has JB Mauney Net Worth Grown Over The Years?
How To Check Balance On VanillaGift.com: A Complete Guide
Unlocking Sukuna Mastery: The Ultimate Guide To Understanding And Achieving Excellence
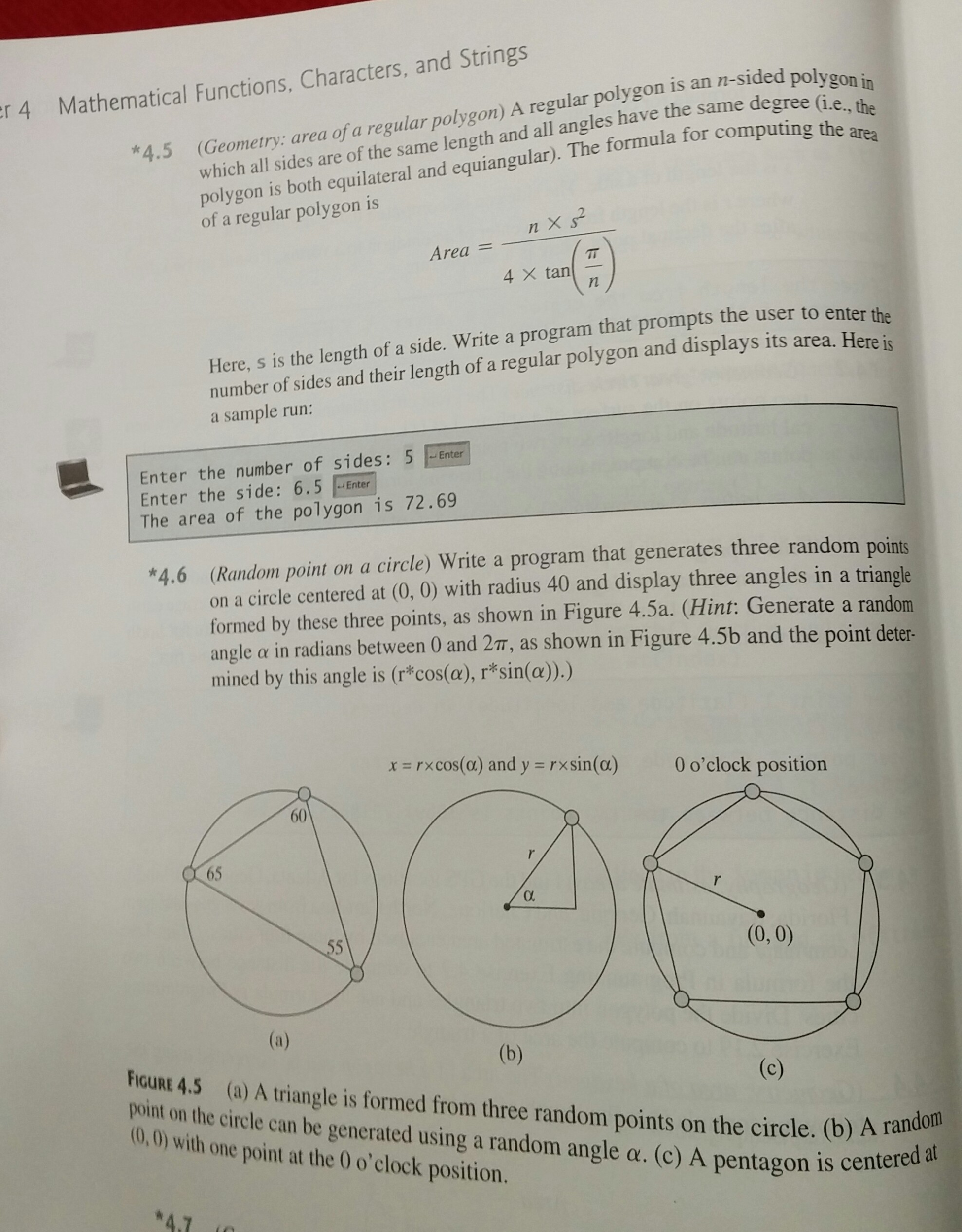
Solved A regular polygon is an nsided polygon in which all
34+ A 9 Sided Polygon Pictures Nosha